Ireland’s first Olympiad was Warsaw 1935, the 6th overall. As with all Olympiads, and indeed many other team tournaments, the definitive reference is the OlimpBase report, and there would be no point in assembling a different report here. However we aim to make the games archive a comprehensive source of Irish games, so the games certainly belong, and have now been added. There are 52 games in all: 43 games by the Irish team (Brian Reilly, James Creevey, T.G. Cranston, J.J. O’Hanlon, and reserve Austin de Búrca) and a further 9 games by C.H.O’D. Alexander (b. Cork, 1909), who was representing Great Britain. (Was this really “Great Britain”, as given by OlimpBase, or “England”? A question for another day.) There were a further 38 games involving these players for which the scores are not available.
Most of these games are available on the ICU web site, of course. But every so often a long-lost game score turns up, and this seems to have happened recently at OlimpBase, which has the game Cranston-Sørenson from board 4 of the Ireland-Denmark match in round 16, played on 28th August 1935. The source isn’t given explicitly but OlimpBase’s “What’s new” page says that 15 games by Danish players were added (2nd April 2010) based on information from P. Skjoldager. (Is this Per Skjoldager, co-author of Chesscafe.com’s 2012 Book of the Year Aron Nimzowitsch 1886-1924? Probably.)
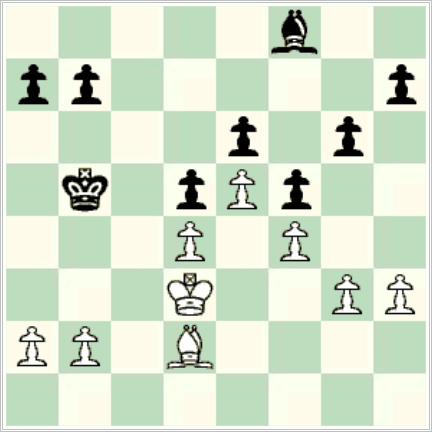The game ended badly for Ireland. In the diagrammed position, it’s Cranston (White) to play, facing considerable difficulties. Cranston played 37. a3? and after 37. … Ka4 38. Kc2 Bxa3! was already lost.
If instead 37. b3 Ba3 38. Kc2 a5 followed by … a4 and … Bb4, it seems White also loses. But after 37. Kc2!?, what is the verdict? The point is that 37. … Kc4 can be met by 38. Be3, planning a4 and b3+ and a perpetual. If 37. … Bb4 38. Be3 Be1 39. g4, it all looks very precarious for White, but where’s the breakthrough? I think White hangs on. Corrections are welcome: can any reader prove that Black can win?